Precalculus by Richard Wright
Are you not my student and
has this helped you?
This book is available
to download as an epub.
Precalculus by Richard Wright
Are you not my student and
has this helped you?
This book is available
to download as an epub.
Blessed are those who mourn, for they will be comforted. Matthew 5:4 NIV
Summary: In this section, you will:
SDA NAD Content Standards (2018): PC.6.1
An instrument company makes guitars and is starting production on a new model. It has a one-time cost of $10,050 to set up the factory production line and materials cost $150 per guitar. The company is going to sell the the guitars for $300 each. How many guitars do they need to sell to break even, where the costs equal the revenue?
To solve this problem, create an equation for costs and one for revenue. This will be a system of equations to be solved. In this lesson, the method will be elimination. Unlike substitution, elimination only works for linear equations.
Linear systems of equations in two-variables produce three possible types of solutions. The lines may intersect in one point. The equations may graph the same line, so all the points on the line are solutions. Or, the line may be parallel and never intersect, so there is no solution. If there is at least one solution, the system is consistent. If there is only one solution, it is independent, but many solutions is dependent because the y-value of the solution depends on the x-value. If there is no solution, the system is inconsistent.
If all the variables are eliminated and the result is
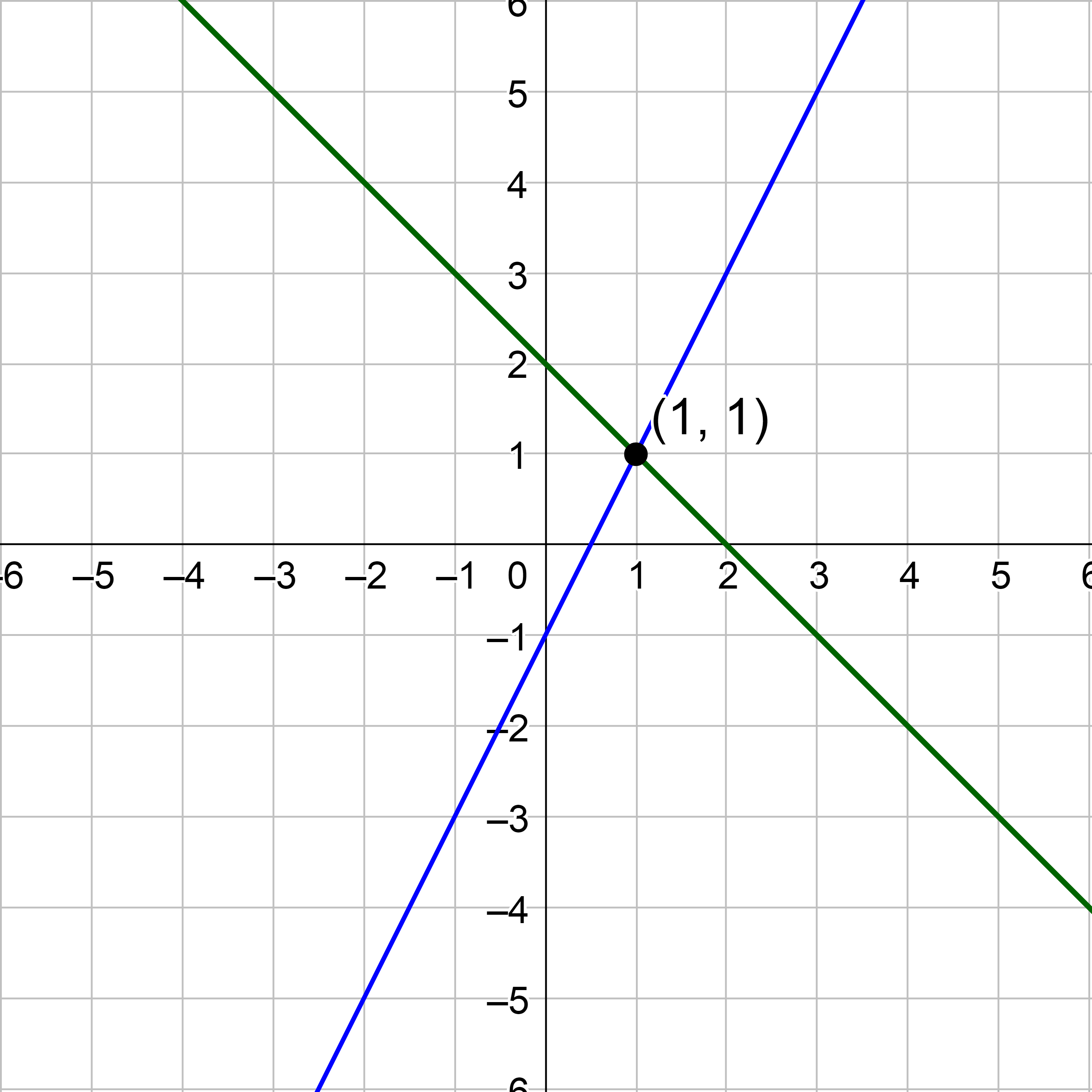
Solve \(\left\{\begin{align} 4x + y &= -3 \\ x - 3y &= 9 \end{align}\right.\) by elimination.
Solution
The system is already written in columns. If the first equation were multiplied by 3, then the coefficients of y would be 3 and −3.
$$ \left\{\begin{align} 3\left(4x + y\right) &= 3\left(-3\right) \\ x - 3y &= 9 \end{align}\right. $$
$$ \left\{\begin{align} 12x + 3y &= -9 \\ x - 3y &= 9 \end{align}\right. $$
Add the equations
$$ \begin{align} 12x + 3y &= -9 \\ + \quad \underline{x - 3y} &= \underline{9} \\ 13x \qquad &= 0 \end{align} $$
x = 0
Back-substitute this into one of the original equations.
4x + y = −3
4(0) + y = −3
y = −3
The solution seems to be (0, −3). Check this by plugging it into both of the original equations.
$$ \left\{\begin{align} 4x + y &= -3 \\ x - 3y &= 9 \end{align}\right. $$
$$ \left\{\begin{align} 4(0) + (-3) &= -3 \\ (0) - 3(-3) &= 9 \end{align}\right. $$
$$ \left\{\begin{align} -3 &= -3 \\ 9 &= 9 \end{align}\right. ✓ $$
The solution is (0, −3), and the system is consistent and independent because there is one solution.
Solve \(\left\{\begin{align} 2x + 3y &= -6 \\ 3x + 5y &= -11 \end{align}\right.\) by elimination.
Solution
The system is already written in columns. If the first equation were multiplied by 3 and the second equation were multiplied by −2, then the coefficients of x would be 6 and −6.
$$ \left\{\begin{align} 3\left(2x + 3y\right) &= 3\left(-6\right) \\ -2\left(3x + 5y\right) &= -2\left(-11\right) \end{align}\right. $$
$$ \left\{\begin{align} 6x + 9y &= -18 \\ -6x - 10y &= 22 \end{align}\right. $$
Add the equations
$$ \begin{align} 6x + 9y &= -18 \\ +\quad \underline{-6x - 10y} &= \underline{22} \\ -y &= 4 \end{align} $$
y = −4
Back-substitute this into one of the original equations.
2x + 3y = −6
2x + 3(−4) = −6
2x − 12 = −6
2x = 6
x = 3
The solution seems to be (3, −4). Check this by plugging it into both of the original equations.
$$ \left\{\begin{align} 2x + 3y &= -6 \\ 3x + 5y &= -11 \end{align}\right. $$
$$ \left\{\begin{align} 2(3) + 3(-4) &= -6 \\ 3(3) + 5(-4) &= -11 \end{align}\right. $$
$$ \left\{\begin{align} -6 &= -6 \\ -11 &= -11 \end{align}\right. ✓ $$
The solution is (3, −4), and the system is consistent and independent because there is one solution.
Solve \(\left\{\begin{align} -3x + 5y &= -21 \\ 2x - 7y &= 25 \end{align}\right.\) by elimination.
Answer
(2, −3); consistent and independent
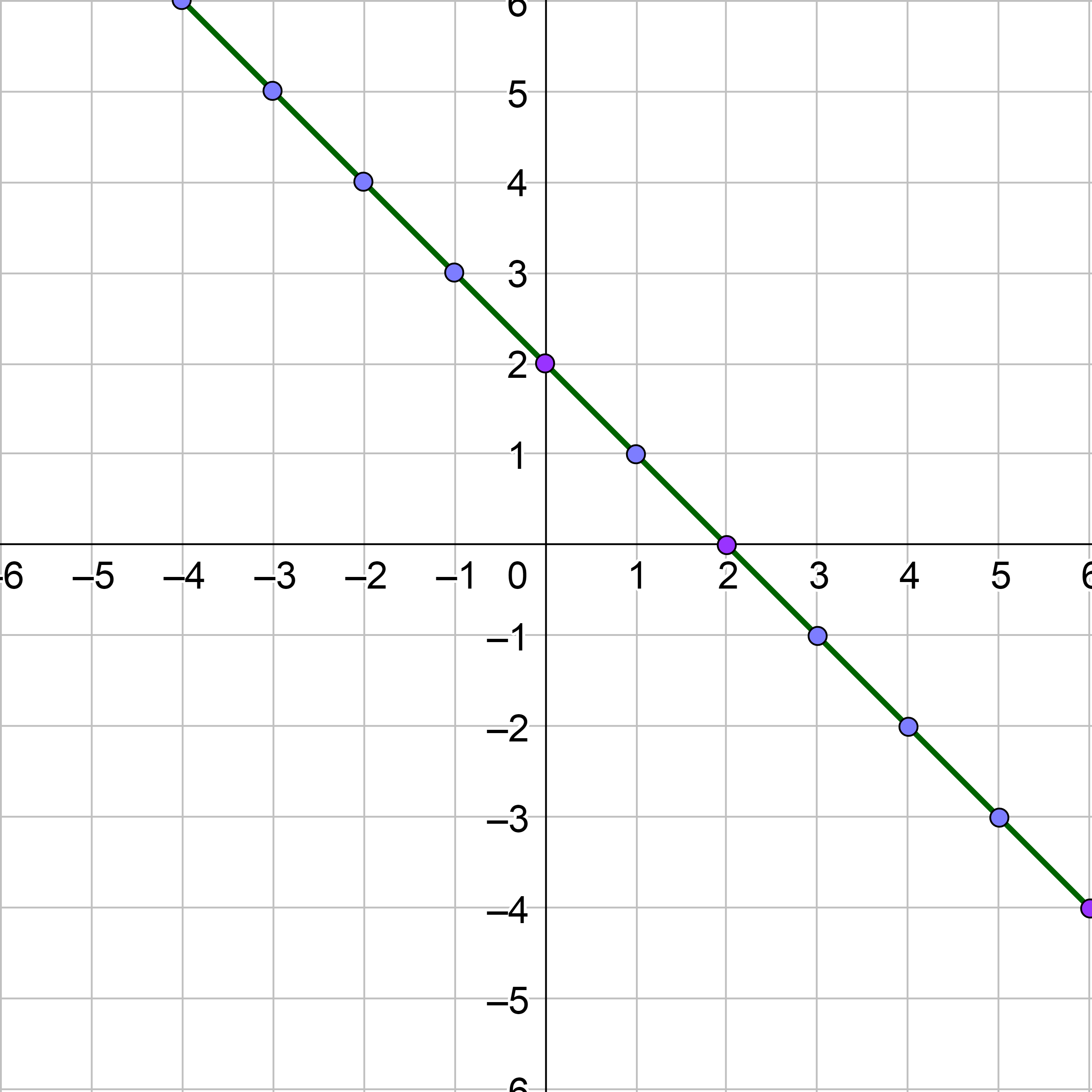
Solve \(\left\{\begin{align} 2x - 6y = 4 \\ x = 3y + 2 \end{align}\right.\) by elimination.
Solution
The system is not written in columns. In the second equation, subtract 3y from both sides to make the columns line up.
$$ \left\{\begin{align} 2x - 6y &= 4 \\ x - 3y &= 2 \end{align}\right. $$
If the second equation were multiplied by −2, then the coefficients of x would be 2 and −2.
$$ \left\{\begin{align} 2x - 6y &= 4 \\ -2\left(x - 3y\right) &= -2\left(2\right) \end{align}\right. $$
$$ \left\{\begin{align} 2x - 6y &= 4 \\ -2x + 6y &= -4 \end{align}\right. $$
Add the equations
$$ \begin{align} 2x - 6y &= 4 \\ +\quad \underline{-2x + 6y} &= \underline{-4} \\ 0 &= 0 \end{align} $$
All the variables were eliminated and a true statement resulted. Thus there are infinitely many solutions described by the equation x − 3y = 2, and the system is consistent and dependent.
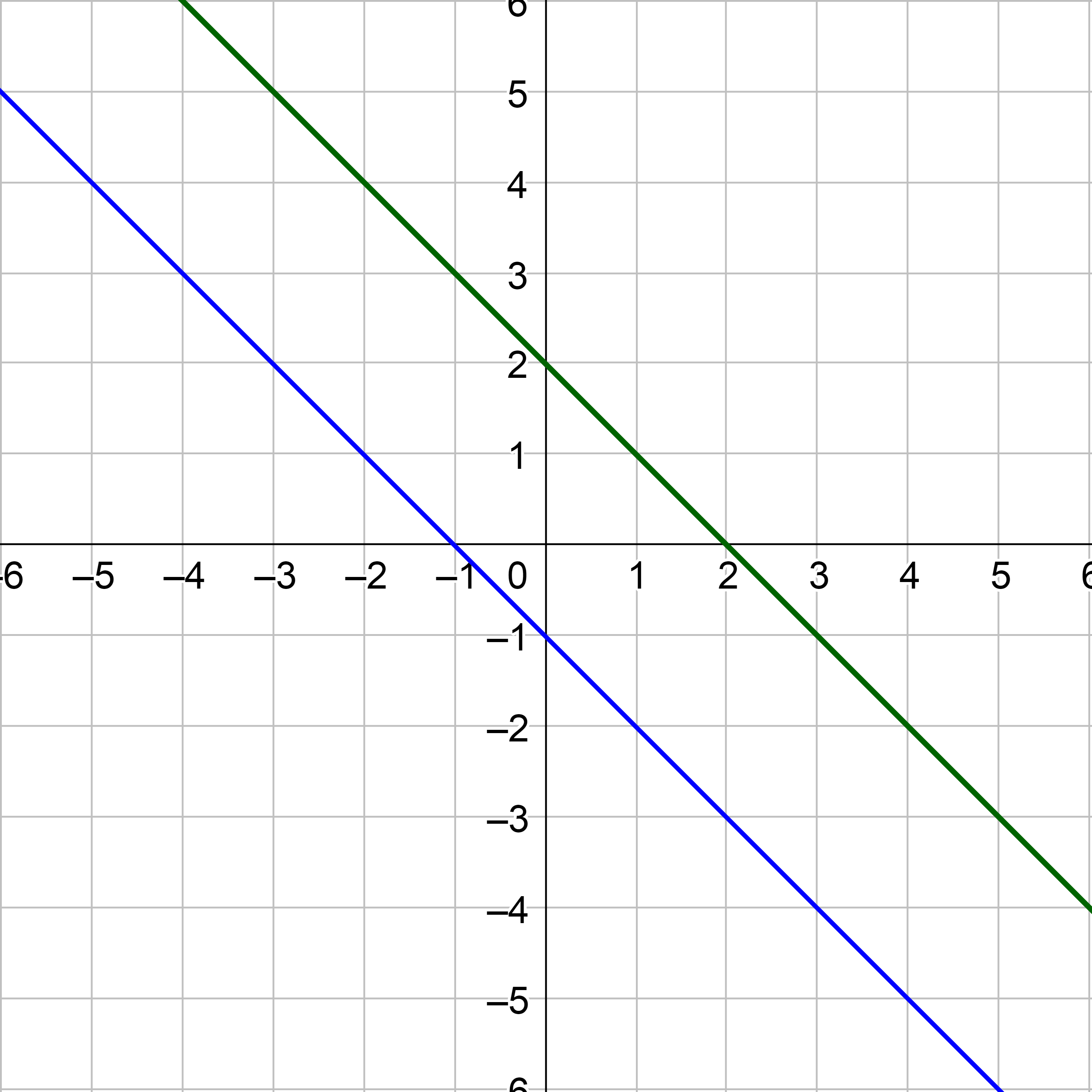
Solve \(\left\{\begin{align} 14x + 21y &= 35 \\ -6x - 9y &= -12 \end{align}\right.\) by elimination.
Answer
No solution; inconsistent
An instrument company makes guitars and is starting production on a new model. It has a one-time cost of $10,050 to set up the factory production line and materials cost $150 per guitar. The company is going to sell the the guitars for $300 each. How many guitars do they need to sell to break even, where the costs equal the revenue?
Solution
Write two equations: one for cost on one for revenue. The costs are C = 10,050 + 150x where x is the number of guitars made. The revenue is the amount of money earned, R = 300x. This question asks for the break even point where cost equals revenue. Replace the C and R with y since they are equal, and write the system of equations.
$$ \left\{\begin{align} y &= 10,050 + 150x \\ y &= 300x \end{align}\right. $$
Rewrite to make nice columns.
$$ \left\{\begin{align} 150x - y &= -10,050 \\ 300x - y &= 0 \end{align}\right. $$
Multiply the top equation by −1 and add.
$$ \begin{align} -150x + y &= 10,050 \\ +\quad \underline{300x - y} &= \underline{0} \\ 150x &= 10,050 \end{align} $$
Solve.
x = 67
The company would need to sell 67 guitars to break even.
If all the variables are eliminated and the result is
Helpful videos about this lesson.
