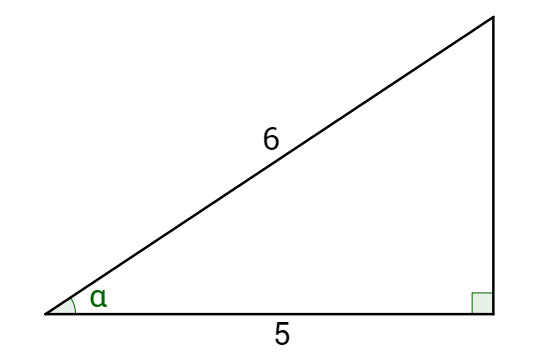
Precalculus by Richard Wright

Previous Lesson Table of Contents Next Lesson

Are you not my student and
has this helped you?

This book is available
to download as an epub.


But the things that come out of a person’s mouth come from the heart, and these defile them. Matthew 15:18 NIV

4-Review

Take this test as you would take a test in class. When you are finished, check your work against the answers. On this assignment round your answers to three decimal places unless otherwise directed.

  1. Sketch the following angles in standard position.
    1. 135°
    2. \(\frac{11π}{6}\)
    3. \(\frac{π}{2}\)
    4. 4.5
  2. Find two coterminal angles—one positive and one negative—of a) \(\frac{2π}{3}\) and b) 420°
  3. Convert to the other angle unit. a) 120° b) 15° c) \(\frac{4π}{3}\) d) \(\frac{π}{10}\)
  4. Find the reference angle in radians. a) \(\frac{5π}{3}\) b) \(\frac{π}{4}\) c) \(\frac{3π}{4}\)
  5. Using the unit circle or special right triangles, evaluate the following. a) \(\cos \frac{π}{3}\) b) \(\tan \frac{3π}{2}\) c) \(\csc \frac{5π}{6}\) d) sec π
  6. A point on a angle α is (3, 7). Evaluate a) sin α b) cos α c) tan α d) sec α.
  7. Given that \(\sin β = \frac{4}{5}\) and β is an acute angle, find a) cos β and b) cot β.
  8. Given that \(\tan θ = −\frac{\sqrt{3}}{3}\) and cos θ > 0; a) what quadrant does θ lie in? b) Evaluate sec θ and c) sin θ.
  9. Consider \(y = 2 \sin\left(πx − \frac{π}{2}\right)\). a) Find the amplitude. b) Find the period. c) Find the phase shift.
  10. Find a function to model this graph.
  11. Find the exact value of \(\cos\left(\sin^{−1} \frac{3}{4}\right)\).
  12. Find the exact value of \(\arcsin\left(\cos π\right)\)
  13. A ship is 10 miles north and 20 miles east of its destination. How far and at what bearing should it sail? (Round to 1 decimal place and use degrees.)
  14. A mass is bouncing on the end of a spring. If its height at t = 0 is 5 cm above equilibrium and it returns to the highest point after 3 seconds, write a function to model the height from equilibrium.
  15. Use the right triangle to evaluate

    1. cot α.
    2. sin α.
    3. sec α.
    4. α (in degrees).

Answers

  2. \(\frac{8π}{3}, −\frac{4π}{3}\); 60°, −300°
  3. \(\frac{2π}{3}\); \(\frac{π}{12}\); 240°; 18°
  4. \(\frac{π}{3}; \frac{π}{4}; \frac{π}{4}\)
  5. \(\frac{1}{2}\); undefined; 2; −1
  6. \(\frac{7\sqrt{58}}{58}; \frac{3\sqrt{58}}{58}; \frac{7}{3}; \frac{\sqrt{58}}{3}\)
  7. \(\frac{3}{5}; \frac{3}{4}\)
  8. IV; \(\frac{2\sqrt{3}}{3}\); \(−\frac{1}{2}\)
  9. 2; 2; \(\frac{1}{2}\)
  10. \(y = \frac{1}{2}\sec\left(2πx\right)\)
  11. \(\frac{\sqrt{7}}{4}\)
  12. \(−\frac{π}{2}\)
  13. 22.4 miles at W 26.6° S
  14. \(y = 5\cos\left(\frac{2π}{3}t\right)\)
  15. \(\frac{5\sqrt{11}}{11}; \frac{\sqrt{11}}{6}; \frac{6}{5}; 33.6°\)