Precalculus by Richard Wright
Are you not my student and
has this helped you?
This book is available
to download as an epub.
Precalculus by Richard Wright
Are you not my student and
has this helped you?
This book is available
to download as an epub.
Blessed are those who hunger and thirst for righteousness, for they will be filled. Matthew 5:6 NIV
Summary: In this section, you will:
SDA NAD Content Standards (2018): PC.5.1
The coefficient of friction is a measure of how slippery a surface is and is used to calculate force of friction. The fundamental trigonometric identities can be used to simplify expressions for the coefficient of friction.
Review the fundamental trigonometric identities in lesson 5-01. This lesson will continue using the identities to simplify trigonometric expressions.
Factor the expression, then use fundamental trigonometric identities to simplify.
csc4 x − cot4 x
Solution
This is a difference of squares. (a2 − b2) = (a − b)(a + b)
csc4 x − cot4 x
(csc2 x − cot2 x)(csc2 x + cot2 x)
The Pythagorean identity, 1 + cot2 x = csc2 x, lets us substitute for csc2 x.
((1 + cot2 x) − cot2 x)((1 + cot2 x) + cot2 x)
1 + 2 cot2 x
Factor and simplify 1 + cos x − cos2 x − cos3 x.
Answer
sin2 x (1 + cos x)
Multiply and use trigonometric identities to simplify.
(3 sin x − 3)(3 sin x + 3)
Solution
Multiply.
(3 sin x − 3)(3 sin x + 3) = 9 sin2 x − 9
Factor out the 9.
9(sin2 x − 1)
A Pythagorean identity relates sin2 x and 1. Solve sin2 x + cos2 x = 1 for sin2 x to get sin2 x = 1 − cos2 x and substitute.
9(1 − cos2 x − 1)
9(−cos2 x)
−9 cos2 x
Simplify (cos x − 1)(cos x + 1).
Answer
−sin2 x
Add or subtract the expressions and use trigonometric identities to simplify.
$$\frac{1}{\cos x + 1} - \frac{1}{\cos x - 1}$$
Solution
Use a common denominator to add the fractions.
$$\frac{1}{\cos x + 1} - \frac{1}{\cos x - 1}$$
$$\frac{\cos x - 1}{\left(\cos x + 1\right)\left(\cos x - 1\right)} - \frac{\cos x + 1}{\left(\cos x + 1\right)\left(\cos x - 1\right)}$$
$$\frac{–2}{\cos^{2} x - 1}$$
Use the Pythagorean identity, sin2 x + cos2 x = 1, solved for cos2 x to substitute. cos2 x = 1 − sin2 x
$$\frac{–2}{1 - \sin^{2} x - 1}$$
$$\frac{–2}{-\sin^{2} x}$$
2 csc2 x
Simplify \(\frac{1}{1 + \sec x} - \frac{1}{1 - \sec x}\)
Answer
−2 csc x cot x
Rewrite the fraction so it is not in fractional form.
$$\frac{1}{\csc x - \cot x}$$
Solution
Multiply the numerator and denominator by the conjugate of the denominator. This will make the denominator have csc2 x and cot2 x which are related by a Pythagorean identity.
$$\frac{1}{\csc x - \cot x}$$
$$\frac{\csc x + \cot x}{\left(\csc x - \cot x\right)\left(\csc x + \cot x\right)}$$
$$\frac{\csc x + \cot x}{\csc^{2} x - \cot^{2} x}$$
Substitute the Pythagorean identity, 1 + cot2 x = csc2 x.
$$\frac{\csc x + \cot x}{\left(1 + \cot^{2} x\right) - \cot^{2} x}$$
csc x + cot x
Rewrite the fraction so that it is not in fractional form: \(\frac{\sin^2 x}{1 - \cos x}\).
Answer
1 + cos x
Use trigonometric substitution to write the algebraic expression as a trigonometric function.
\(\sqrt{16 - x^{2}}\); x = 4 sin θ
Solution
Substitute the trigonometric expression for x.
$$\sqrt{16 - x^{2}} = \sqrt{16 - \left(4 \sin θ\right)^{2}}$$
$$\sqrt{16 - 16 \sin^{2} θ}$$
$$\sqrt{16\left(1 - \sin^{2} θ\right)}$$
The Pythagorean identity, sin2 x + cos2 x = 1, relates sin2 x and 1. Solve this for sin2 x and substitute. sin2 x = 1 − cos2 x
$$\sqrt{16\left(1 - \left(1 - \cos^{2} θ \right)\right)}$$
$$\sqrt{16 \cos^{2} θ }$$
4 cos θ
Use trigonometric substitution to write the algebraic expression as a trigonometric function.
$$\sqrt{4 + x^2}; x = 2\cot α$$
Answer
2 csc α
Reciprocal Identities
| \(\sin u = \frac{1}{\csc u}\) | \(\csc u = \frac{1}{\sin u}\) |
| \(\cos u = \frac{1}{\sec u}\) | \(\sec u = \frac{1}{\cos u}\) |
| \(\tan u = \frac{1}{\cot u}\) | \(\cot u = \frac{1}{\tan u}\) |
Quotient Identities
| \(\tan u = \frac{\sin u}{\cos u}\) | \(\cot u = \frac{\cos u}{\sin u}\) |
Pythagorean Identities
| \(\sin^{2} u + \cos^{2} u = 1\) |
| \(\tan^{2} u + 1 = \sec^{2} u\) |
| \(1 + \cot^{2} u = \csc^{2} u\) |
Even/Odd Identities
| Even | |
| cos(–u) = cos u | sec(–u) = sec u |
| Odd | |
| sin(–u) = –sin u | csc(–u) = –csc u |
| tan(–u) = –tan u | cot(–u) = –cot u |
Cofunction Identities
| \(\sin \left(\frac{π}{2} - u\right) = \cos u\) | \(\cos \left(\frac{π}{2} - u\right) = \sin u\) |
| \(\tan \left(\frac{π}{2} - u\right) = \cot u\) | \(\cot \left(\frac{π}{2} - u\right) = \tan u\) |
| \(\sec \left(\frac{π}{2} - u\right) = \csc u\) | \(\csc \left(\frac{π}{2} - u\right) = \sec u\) |
Helpful videos about this lesson.
If an object slides down an inclined surface at a constant speed, the force of friction has to equal the component of the object's weight pulling it down the surface. The equation becomes
μW cos θ = W sin θ
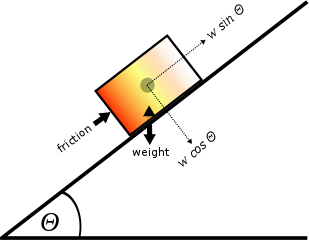
where μ is the coefficient of friction, W is the weight, and θ is the incline of the surface. Solve the equation for μ and use fundamental trigonometric identities to simplify the expression.